Travis Norsen
(1975?-)
Travis Norsen is a professor of physics at Smith College. He authored the 2017 textbook
Foundations of Quantum Mechanics: An Exploration of the Physical Meaning of Quantum Theory, which contains a critical analysis of
Bell's Theorem.
Norsen describes Bell's description of locality in his later years using the CHSH derivation rather than Bell's original "ad hoc" model.
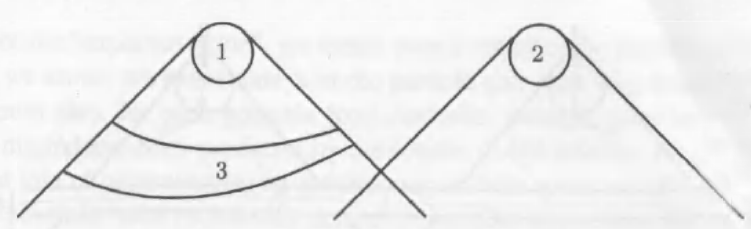
Fig. 8.4 Space-time regions relevant to Bell’s formulation of local causality. Bell writes: “Full specification of what happens in 3 makes events in 2 irrelevant for predictions about 1 in a locally causal theory”
Ref. "La Nouvelle Cuisine," in Speakable and Unspeakable in Quantum Mechanics, Cambridge, p.240
But Norsen then explicitly shows how a
common cause from the initial entanglement is still in the past light cone of the "
separated" measurements at A and B.
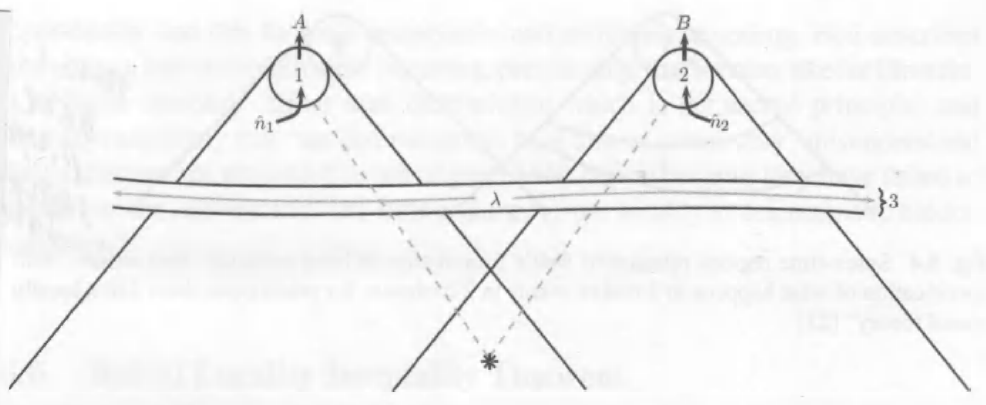
Fig. 8.5 Space-time diagram for the Bell experiment. The particle pair is emitted at the “flash’' at the bottom of the diagram; world-lines for the two individual particles flying apart in opposite directions are represented by the gray dashed lines. The (large!) region 3 encompasses both particles at some intermediate time and shields the two measurement regions, 1 and 2. from their overlapping past light cones in the way that is required in Bell's formulation of locality.
It is not clear how Bell's "shield" could prevent common causes coming from the initial entanglement.
In our explanation of two-particle entanglement the
first step is the formation of the
spherical symmetric singlet state.
The
second step is the conservation of the total spin angular momentum (and its spherical symmetry), which is a
constant of the motion as the entangled particles fly apart.
The
third step is the conserved
spherical symmetry. As long as the two measurement devices are set at a (pre-agreed upon) same angle, they will have planar symmetry which bisects the spherical symmetry. Their shared measurement angle can then be arbitrary and the interactions will still produce
perfectly correlated results.
For a summary of the three steps see our page
How a Common Cause, a Constant of the Motion, and Spherical Symmetry Can Explain Einstein's "
Spooky Action at a Distance"
The argument by
David Bohm1 that a common cause would require particles spins to be defined in all three x, y, z directions (which of course is impossible) and by
N. David Mermin2 that photons must be polarized in all directions, are not correct.
The fundamental requirement for
perfectly correlated measurement results is that the two-particle wave function has
no preferred direction and a single arbitrary direction is chosen for measurements at A and B.
1. Bohm, D. and Y. Aharonov, Discussion of Experimental Proof for the Paradox of Einstein, Rosen, and Podolsky, Physical Review vol.108, no.4, Nov.15, 1957
2. D. Mermin, Boojums All The Way Through (1990), Cambridge University Press, p.110
Normal |
Teacher |
Scholar