The laws of nature, except the second law of thermodynamics, are symmetric in time. Reversing the time in the equations of motion simply describes everything going backwards. The second law is different. Entropy must never decrease in time.

Arthur Stanley Eddington coined the term "Arrow of Time" in his 1927 book The Nature of the Physical World. He connected "Time's Arrow" to the one-way direction of increasing entropy required by the second law of thermodynamics.

If the laws of nature are time symmetric, perhaps the arrow of time is to be found in the "initial" conditions, although this may be a circular concept, since "initial,"current," and "final" states are all defined with respect to time. Nevertheless, most physicists have assumed the universe must have begun in a highly ordered state and it has been "running down" (entropy or disorder increasing) ever since.

Would time be reversed if we could make the entropy decrease? That is unlikely, since entropy decrease anywhere (creating negative entropy or negentropy, a term coined by Leon Brillouin) must be accompanied by an increase elsewhere, to satisfy the second law. Otherwise we could use the local reduction in the entropy to build a perpetual motion machine.

Put another way, if we could reverse the time, would entropy decrease? What would time reversal really mean? For Aristotle, time was a measure of motion and change. If we could reverse the motions of every material body, would that make the entropy decrease? Ludwig Boltzmann agreed that it might, for a while, but that it could not return to a highly ordered original state. [see Layzer's Perfume Bottle.]

The fundamental question of information philosophy is cosmological and ultimately metaphysical. What is the process that creates information structures in the universe?

Given the second law of thermodynamics, which says that any system will over time approach a thermodynamic equilibrium of maximum disorder or entropy, in which all information is lost, and given the best current model for the origin of the universe, which says everything began in a state of equilibrium some 13.75 billion years ago, how can it be that living beings are creating and communicating new information every day? Why are we not still in that state of thermal equilibrium?

It is perhaps easier for us to see the increasing complexity and order of information structures on the earth than it is to notice the increase in chaos that comes with increasing entropy, since the entropy is radiated away from the earth into the night sky and away to the cosmic microwave background sink of deep space.

David Layzer is a Harvard cosmologist who in the early 1970's made it clear that in an expanding universe the entropy would increase, as required by the second law of thermodynamics, but that the maximum possible entropy of the universe might increase faster than the actual entropy increase. This would leave room for an increase of order or information at the same time the entropy is increasing!

Layzer pointed out that if the equilibration rate of the matter (the speed with which matter redistributes itself randomly among all the possible states) was slower than the rate of expansion, then the "negative entropy" or "order" (defined as the difference between the maximum possible entropy and the actual entropy) would also increase. Claude Shannon identified this negative entropy with information, though visible structural information in the universe may be less than this "potential" for information.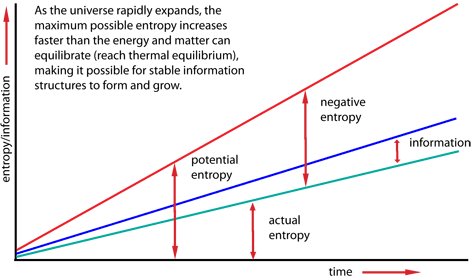

Layzer called the direction of information increase the "historical arrow."

In a 1975 article for Scientific American called The Arrow of Time, Layzer wrote:

the complexity of the astronomical universe seems puzzling.

This is the fundamental question of information philosophy

Isolated systems inevitably evolve toward the featureless state of thermodynamic equilibrium. Since the universe is in some sense an isolated system, why has it not settled into equilibrium? One answer, favored by many cosmologists, is that the cosmological trend is in fact toward equilibrium but that too little time has elapsed for the process to have reached completion... I shall argue that this view is fundamentally incorrect. The universe is not running down, and it need not have started with a marked degree of disequilibrium; the initial state may indeed have been wholly lacking in macroscopic as well as microscopic information.

Suppose that at some early moment local thermodynamic equilibrium prevailed in the universe. The entropy of any region would then be as large as possible for the prevailing values of the mean temperature and density. As the universe expanded from that hypothetical state the local values of the mean density and temperature would change, and so would the entropy of the region. For the entropy to remain at its maximum value (and thus for equilibrium to be maintained) the distribution of energies allotted to matter and to radiation must change, and so must the concentrations of the various kinds of particles. The physical processes that mediate these changes proceed at finite rates; if these "equilibration" rates are all much greater than the rate of cosmic expansion, approximate local thermodynamic equilibrium will be maintained; if they are not, the expansion will give rise to significant local departures from equilibrium.

This is the Layzer's seminal theory of the growth of order in the universe

These departures represent macroscopic information; the quantity of macroscopic information generated by the expansion is the difference between the actual value of the entropy and the theoretical maximum entropy at the mean temperature and density.

(Scientific American, December, 1975, p.68)

In his 1989 book The Emperor's New Mind, Roger Penrose speculated on the connection between information, entropy, and the arrow of time.

Recall that the primordial fireball was a thermal state — a hot gas in expanding thermal equilibrium. Recall, also, that the term 'thermal equilibrium' refers to a state of maximum entropy. (This was how we referred to the maximum entropy state of a gas in a box.) However, the second law demands that in its initial state, the entropy of our universe was at some sort of minimum, not a maximum!

What has gone wrong? One 'standard' answer would run roughly as follows:

True, the fireball was effectively in thermal equilibrium at the beginning, but the universe at that time was very tiny. The fireball represented the state of maximum entropy that could be permitted for a universe of that tiny size, but the entropy so permitted would have been minute by comparison with that which is allowed for a universe of the size that we find it to be today. As the universe expanded, the permitted maximum entropy increased with the universe's size, but the actual entropy in the universe lagged well behind this permitted maximum. The second law arises because the actual entropy is always striving to catch up with this permitted maximum.

(The Emperor's New Mind, p.328-9)

Penrose's "standard" answer is a clear reference to the pioneering work of Harvard cosmologist David Layzer, especially his 1975 Scientific American article "The Arrow of Time." Layzer explained the the growth of order in the universe as the maximum possible entropy of the expanding universe increasing faster than the actual entropy, because the equilibration rates for matter and radiation are slower than the expansion rate. In 1945, John Wheeler and his student Richard Feynman attempted to symmetrize Maxwell's equations for electromagnetic fields.

We can define a cosmological direction of time as the direction in which the universe is expanding. There are excellent reasons for seeing this as the most fundamental of all arrows, even the one driving some of the others. Without expansion, a static universe would settle into thermal equilibrium and there would be no changes. There would he no entropy increase, to show Eddington's thermodynamic arrow. There would be no information increase, as seen in Layzer's historical arrow.